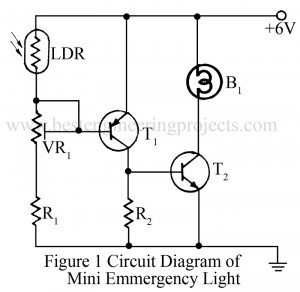
There are various type of emergency light circuits. Every circuit has its own importance and utility. But size, cost and efficiency of the circuit are some of the main feature of interest. A very simple circuit of mini emergency light is given here. In normal daylight or electric light, the LDR offer very low resistance. So the positive voltage is applied to the base of transistor T1, making it reverse biased. Therefore, T1 does not conduct in presence of light. As transistor T2 also is reverse biased it does not…
Read MoreAutomatic Cut off Power Supply
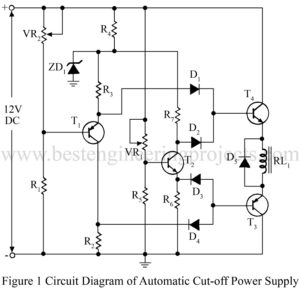
We are aware that when the line voltage becomes more than 250 volts and less than 160 volts, it can damage our TV sets, refrigerators, etc. Hence, we use voltage stabilizers which are costly. The circuit Automatic Cut-off Power Supply described here is low-cost. The assembling cost comes to around $1 only. If the voltage exceeds the mentioned range it automatically cuts off the supply so that the life of the TV set and other devices can be extended. Circuit Description of Automatic Cut off Power Supply The common point of…
Read MoreAutomatic Time Indicator for Telephone
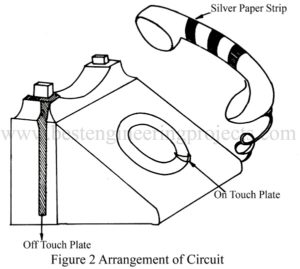
The circuit Automatic Time Indicator for Telephone presented here provides an audible warning 2.5 minutes after dialing the telephone. The circuit does not make use of telephone line for its operation. Circuit Description of Automatic Time Indicator for Telephone The circuit consists of three 555 ICs. It can be divided into three parts- touch switch using IC1, timer using IC2 and oscillator using IC3. The on touch plate is in the form of a wire, the insulation of which is attached to the stopper on the telephone dial. The off touch…
Read MoreElectronic Ballast Circuit
The fluorescent tube-light requires additional gear such as the copper ballast and starter for normal operation. These two are required to provide the initial high voltage for ionization and thereafter to limit the current through the tube to safe values. It has been observed that the illumination efficiency of the tube-light when excited by high frequency power source is higher than that when excited at the 50Hz line frequency. Moreover, the power factor and the efficiency of the bulky copper ballasts are poor. Hence, electronic ballasts circuit were developed to…
Read MoreLibrary Book Info System (LBIS)
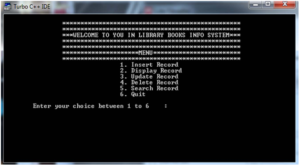
The project ‘Library Book Info System (LBIS)’ is going to develop for College has a good library enriched with a lot of books. The prime objective of the project is to fulfill the requirements of college library. This project has been developed to replace the existing manual system for keeping books records with computerized automated books record processing system. This project will automatically reduce the human efforts, time and cost and increases efficiency and accuracy of storing books records. The library staffs can get the right information in time and…
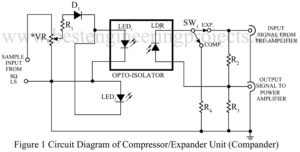
Read MoreExpander Compressor Unit Circuit | Compander
The compression and expansion are two important techniques used by audio engineers during recording and reproduction of program material. The compression of dynamic range of program material (records, speech or musical broadcasting) permits maintaining constantly high modulating level while the expansion, when used with the reproduction of compressed material, restores the dynamic range and creates a ‘live’ music. Thus, we are created simple and basic project Expander Compressor Unit Circuit. Circuit Description of Expander Compressor Unit Circuit | Compander Creating these effects is costly and complex, and beyond the scope of amateur…
Read MoreSoil Stabilization | Soil Stabilization Method
The process of improving the engineering properties of soils is called soil stabilization. It is the simplest method of soil stabilization. By soil stabilization, the soil becomes more stable by the reduction in the permeability and compressibility and by the increase in shear strength. With the increase in soil stabilization properties the bearing capacity of soil is increased significantly. The various method of soil stabilization includes: Mechanical Stabilization Cement Stabilization Lime Stabilization Bitumen Stabilization Stabilization by Geo-textiles Mechanical Stabilization | Soil Stabilization | Soil Stabilization Method In this method changing the…
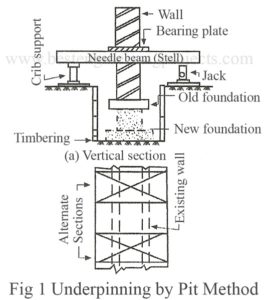
Read MoreUnderpinning | Underpinning Methods and Purpose
What is underpinning? Underpinning is a process of placing a new foundation under an existing one or strengthening an existing foundation. What are the Purpose of Underpinning? The underpinning is done to serve the following purposes. To strengthen a shallow foundation when a building with deep foundation is to be constructed adjoining it. To strengthen an existing foundation, which has suffered from cracks and settlements To deepen an existing foundation. To construct a basement under an existing building. What the Underpinning Methods? There are two underpinning Methods. They are: Pit…
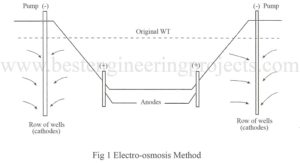
Read MoreDrainage by Electro – Osmotic Method
Electro-osmotic method is used when co-efficient of permeability lies between 10-7 cm/see to 10-5 cm/sec. In electro-osmotic method, two electrodes are driven into the saturated cohesive soil. The cathode is made out of steel in the form of a well point or a tube. A steel rod or a pipe or a sheet piling is made to serve as anode as shown in Fig.1. In general the surface of soil is negative charged and is surrounded by polar water and ions of other elements. When a current is passed through…
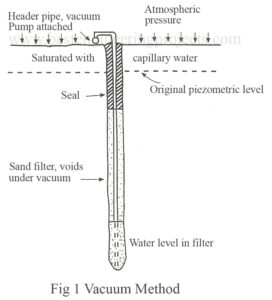
Read MoreDrainage by Vacuum Method
If the effective size of the soil to be drained is less than 0.05 mm, well points cannot be used because capillary forces prevent flow. In order to drain such soils suction head in excess of capillary head is to be applied i.e. by vacuum method. The typical soils of such nature are sands and silty sands. These soils possesses, coefficient of permeability between 1 ×10-3 to 1 ×10-5 cm/sec. Drainage in such cases can be made efficient by the use of vacuum well points as in Fig.1. Vacuum well…
Read More