Bearing Capacity Analysis | Pauker – Rankine Method

Bearing Capacity Analysis | Pauker – Rankine Method

The methods suggested by Pauker Rankine Method are based on theory of classical earth pressure. This was postulated by Pauker a military engineer in 1850 and then modified by Bell in 1915.

Pauker’s theory is only applicable to sands. Bell extended this theory for cohesive soils. Both the theories have not taken into account the width of the foundation. Since the equation has been developed based on Rankine’s earth pressure theory, the theory is called as Rankine’s theory for the determination of the bearing capacity. The theory is too approximate and does not have any practical use. This has been described only for the academic interest.

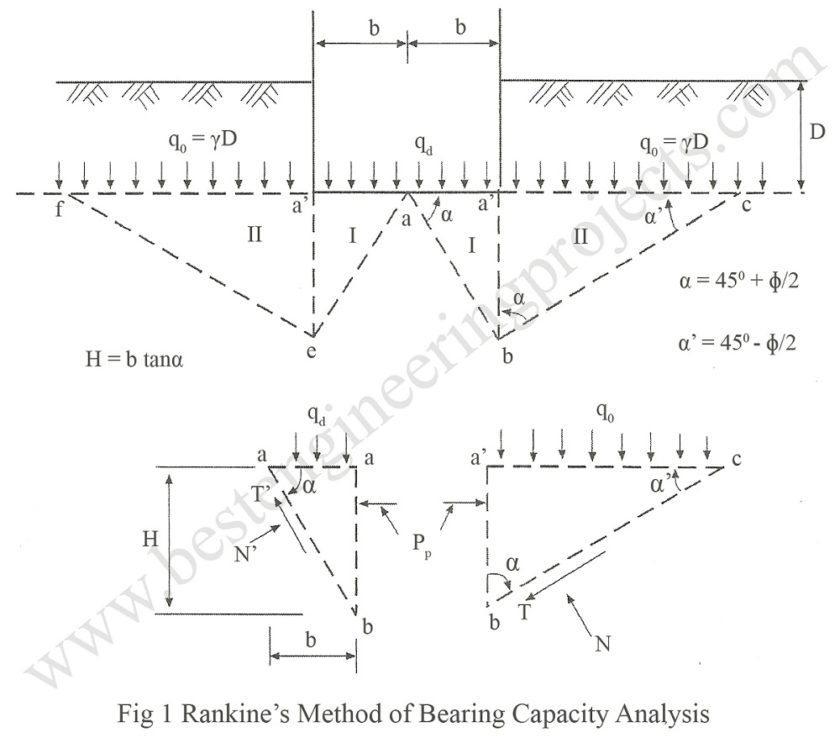
A foundation having width 2b placed at a depth D is shown in Fig.1. The failure surfaces are assumed as broken lines bounded by planes abc and aef. They are symmetrical about the center of the footing. The failure planes are assumed to consist of two wedges.

Wedge I is a Rankine’s active wedge. This is pushed downward by the load q_d acting on the base aa’ of the footing. As a result of downward pressure the vertical face of the wedge will be pushed outwards.

Wedge II is the Rankine’s passive wedge. The pressure acting on the vertical face in wedge II is same as horizontal pressure in wedge I, As a result of pushing of the soil across the face the soil in wedge II gets lifted upwards. If the footing is founded at depth D, from the ground surface the equivalent surcharge will resist the push up resulted by wedge I.

The derivation can be made by starting from the wedge II. The soil in the wedge gets pushed up. This is resisted by the passive earth pressure P_p acting on the face a’b. The surcharge on the face a’c is:

Referring to the fig.1. we have,

   ———- (1)

The soil in the wedge aba’ slides down along the surface ab. The vertical side a’b of the wedge I may be considered as a retaining wall, which moves upward. The total active earth pressure P_a acting at is same as the passive earth pressure P_p on in Wedge II. Then,

P_a = q_dK_a   ———- (2)

  But, P_a = P_p

Therefore,  q_dK_a = q₀K_p

  Then,  q_d = q_oK_p²

Or, q_d = q₀N_q

Where,

   ———- (3)

The above Eqs.(1) and (2) shows that the equation neither considers the width of foundation nor the unit weight of the soil. When the footing rests at the surface D = 0 and hence q_d = 0.This does not address the reality. It is valid for D > 0 but very conservative.