Antilogarithmic Amplifier | Derivation

Antilogarithmic amplifier is one whose output is antilogarithmic (exponential) of input. Like logarithmic amplifier, antilogarithmic is also a non-linear amplifier.

Antilogarithmic Amplifier using Single Transistor

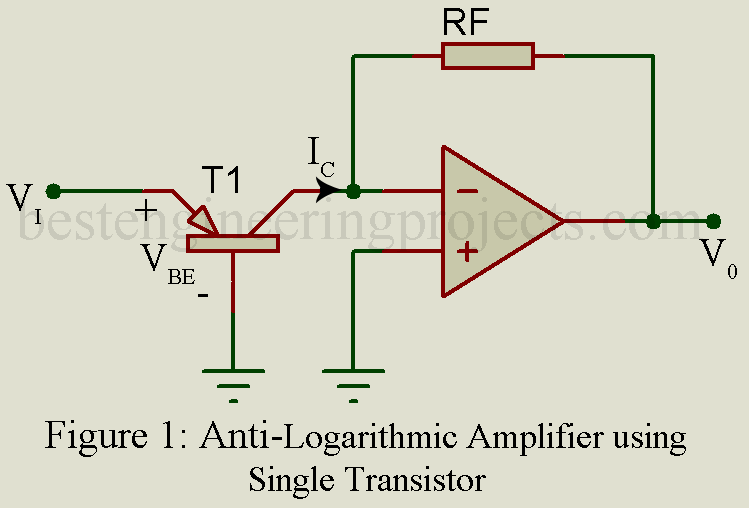
The circuit arrangement for Antilogarithmic amplifier is illustrated in figure 1. Here a general purpose NPN transistor is connected to inverting input of op-amp. A resistor is connected in feedback path. The output is depending upon output current of transistor and feedback resistor.

Now for diagram, output voltage can be written as

$V_0 = -R_f \times I_C$ …..(1)

Where, I_s = Saturation current

V_T = Thermal Voltage

$I_C = I_s \times (e^{\dfrac{V_{BE}}{V_T}-1})$

As we know that, $e^{\dfrac{V_{BE}}{V_T}}>>1$

Thus, we can write $I_C = I_{s} \times e^{\dfrac{V_{BE}}{V_T}}$

Now putting the value of collector current of transistor I_C in equation 1. The output voltage expression becomes

$V_O = -R_f \times I_s \times e^{\dfrac{V_{BE}}{V_T}}$

From the figure we can also conclude that transistor base emitter voltage (V_BE) is equivalent input voltage V_ii.e. $V_{BE} = V_i$

Thus, we can also write output voltage in contest of input voltage V_i

$V_0 = -R_f \times I_s \times e^{\dfrac{V_i}{V_T}}$

Antilogarithmic Amplifier using Matched Diode

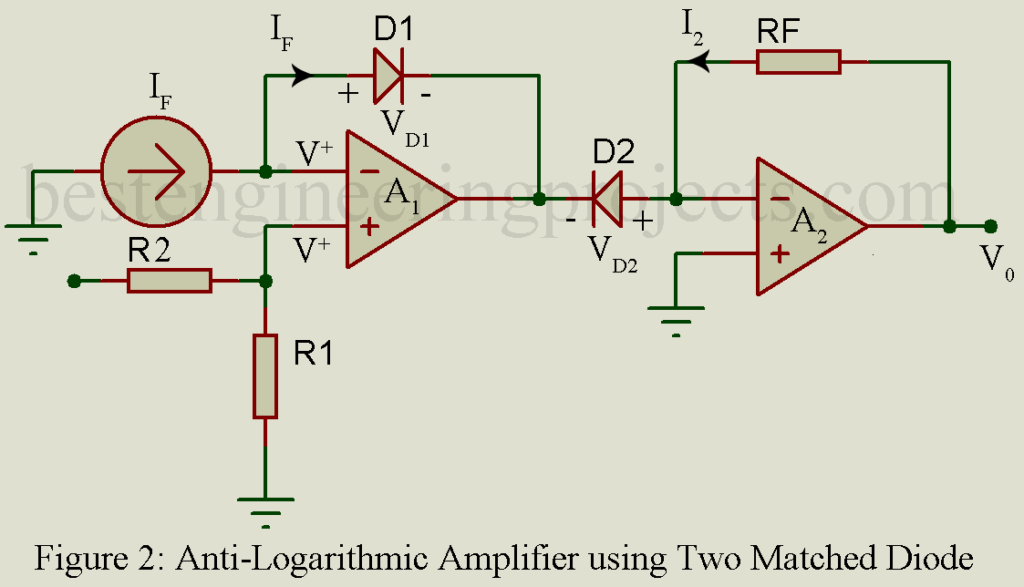
The figure of anti-logarithmic amplifier using matched diode is shown in figure below. Two matched diodes are used here, where one diode (D₁) is connected in feedback path and second diode (D₂) is connected to inverting input of op-amp (A2) in reverse bias mood as shown in figure 2.

Voltage at inverting pin of op-amp (A1) is potentially equal to voltage at non-inverting input of op-amp i.e. inverting input voltage = non-inverting input voltage = V⁺ say. Thus, we can write

$V^+ = \dfrac{R_1}{R_1 + R_2}\times V_s$ ……(2)

Now, we can use KVL in order to solve for V⁺ voltage.

$V^+ -V_{D1} + V_{D2} = 0$

$V^+ = V_{D1} -V_{D2}$ …..(3)

Now, solving for diode

$I_D = I_s \times (e^{\dfrac{V_D}{\eta V_T}}-1)$

Where, $\eta = material constant$

We know that $V^{\dfrac{V_D}{\eta V_T}} >> 1$

Thus, we can write

$I_D = I_s \times e^{\dfrac{V_D}{\eta V_T}}$

Output voltage of diode V_D can be written as,

$V_D = \eta \times V_T \times ln(\dfrac{I_D}{I_s})$

Now, we can calculate individual diode voltage i.e. V_D1 and V_D2.

$V_{D1} = \eta_1 \times V_{T1} \times ln(\dfrac{I_f}{I_{s1}})$

$V_{D2} = \eta_2 \times V_{T2} \times ln(\dfrac{I_2}{I_{s2}})$

Assuming both diode and matched thus material constant, thermal voltage of diode and saturation current of diode is also same. Thus, we can write

$\eta_1 = \eta_2 = \eta$

$V_{T1} = V_{T2} = V_T$

$I_{s1} = I_{s2} = I_s$

From equation 3, we can write

$V^+ = \eta \times V_T \times ln(\dfrac{I_f}{I_2})$

$= -\eta \times V_T \times ln(\dfrac{I_2}{I_f})$

$I_2 = \dfrac{V_0}{R_f}$

Putting the value of I₂ in equation V⁺

$V^+ = -\eta \times V_T \times ln(\dfrac{V_o}{R_f \times I_f})$ …..(4)

Now from equation 2 and 4 we can write

$\dfrac{R_1}{R_1 + R_2} \times V_s = -\eta \times V_T ln(\dfrac{V_o}{R_f \times I_f})$ $V_o = I_f \times R_f \times exp[\dfrac{-V_s}{\eta \times V_T} \times \dfrac{R_1}{R_1 + R_2}]$

Temperature sensitive factor $\eta \times V_T$ can be eliminated by adjusting voltage divider also temperature sensitive in such a way that their effect get can canceled.

Antilogarithmic Amplifier using Two Matched Transistors

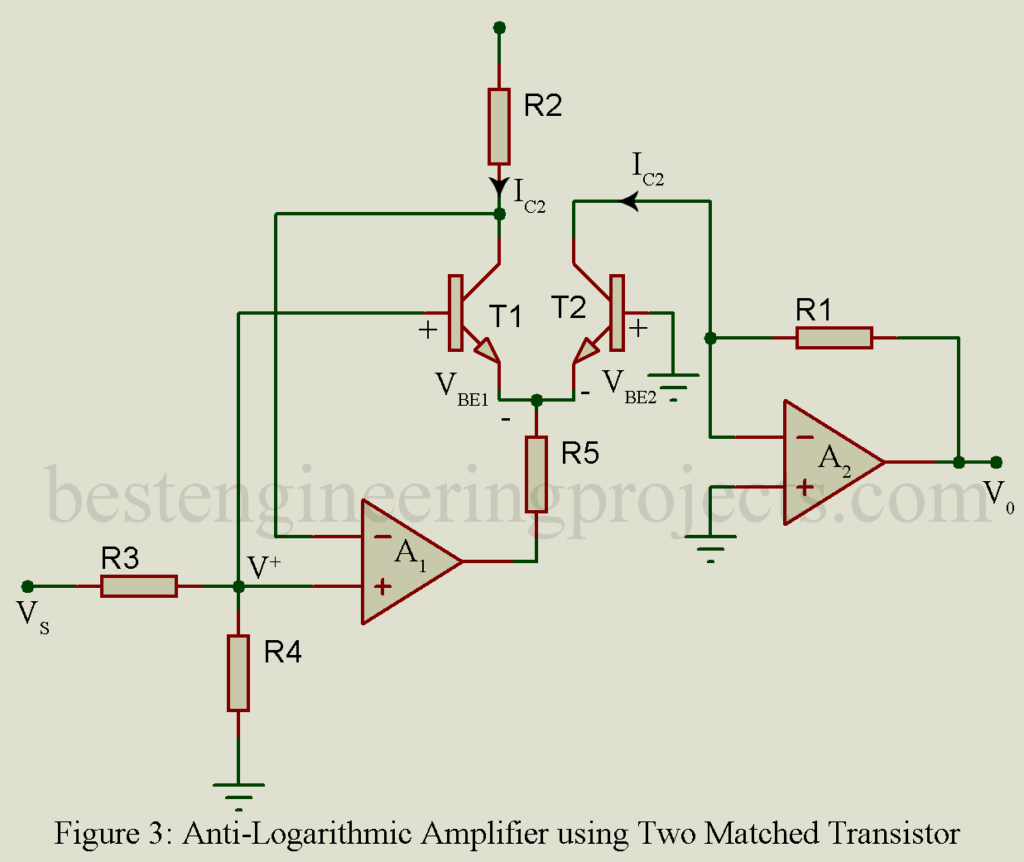
The figure of anti-logarithm amplifier is shown in figure 3. Two matched transistors is used here as shown in figure, where input is given to the non-inverting amplifier pin of first op-amplifier A1.

Now form figure,

$V^+ = \dfrac{R_4}{R_3 + R_4} \times V_s$ ……(5)

$V^+ -V_{BE1} + V_{BE2} = 0$

$V^+ = V_{BE1} -V_{BE2}$ …..(6)

For transistor,

$I_c = I_s \times (e^{\dfrac{V_{BE}}{V_T}}-1)$

We know that, $e^{\dfrac{V_{BE}}{V_T}}>>1$

$I_c = I_s \times e^{\dfrac{V_{BE}}{V_T}}$

$V_{BE} = V_T \times ln(\dfrac{I_c}{I_s})$

Now, we can drive base emitter voltage of each transistor.

Base emitter voltage of 1^st transistor V_BE1 can be written as

$V_{BE1} = V_{T1} \times ln(\dfrac{I_{c1}}{I_{s1}})$

Base emitter voltage of 2^nd transistor can be written as

$V_{BE2} = V_T \times ln(\dfrac{I_{c2}}{I_{s2}})$

Assuming both transistors are matched. Thus, thermal voltage of 1^st transistor will be same to thermal voltage of 2^nd transistor and saturation current of 1^st transistor will be equal to saturation current of 2^nd transistor. i.e.

$V_{T1} = V_{T2} = V_T$ ;

$I_{s1} = I_{s2} = I_s$

From figure we can write,