Prandtl and Bell Theory on Bearing Capacity Analysis

Engineeering Projects

Prandtl and Bell Theory on Bearing Capacity Analysis: Prandtl 1920 developed an equation based on his study of penetration of long hard metal puncher into softer materials for computing the ultimate bearing capacity.

Assumptions made by Prandtl and Bell Theory on Bearing Capacity Analysis

  • The material is softer, homogeneous and isotropic.
  • The material is weightless and possesses only friction and cohesion.
  • The problem is two dimensional
  • The base of the puncher is smooth.
  • The material behaves as a rigid body.
  • The volume change will be Zero.
  • The resulting deformation will be a plastic deformation.

prandtl's failure surface

Failure mechanism – The mechanisms of failure of soil under plastic equilibrium are:

  1. Failure occurs along definite slip surfaces and symmetrical with respect to the centerline of the puncher or footing in the case of soil for symmetrical vertical loading.
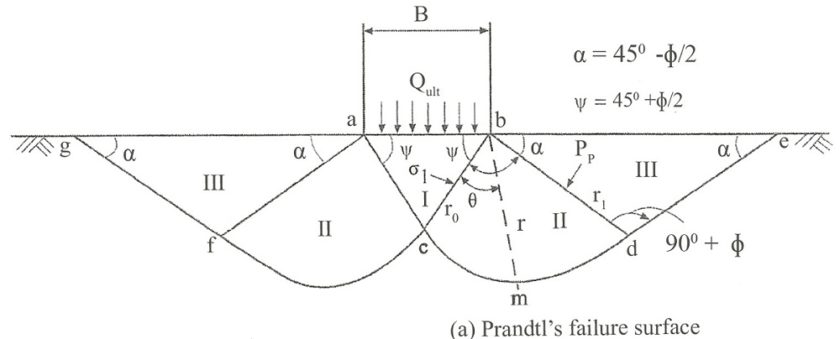
  2. Under ultimate load Qult acting on the footing of width B as shown in Fig(a), a triangular wedge of soil abc is formed below the footing and pushed down as a rigid body. The stresses acting on abc will be in active stage.
  3. Due to gradual downward push of the wedge abc, the soil mass on its left and right will be pushed outward and upward. The failure takes place along the slip surfaces bcde and acfg. These surfaces comprise triangular wedges bde and afg, and sectors bcd and acf.
  4. The mass of soil will be in a plastic state above the failure surface gfcde and in elastic state below the surface.
  5. The failure surfaces can be divided into three Zones I to III as Shown in the Fig.(a). Zone I will be in active state, Zone II in radial shear and Zone III in plastic state.
  6. The lower boundaries of zone II are parts of logarithmic spirals with their poles coinciding with the corresponding edge of the footing. The straight lines de and fg are tangential to spirals at points d and f respectively and meet the horizontal surface at angles of (45^0-\dfrac{\phi}{2})

Bell’s Theory on Bearing Capacity Theory

Cohesive soil – Bell extended the theory of Rankine and Pauker for cohesive soil.

For this case we can write,

P_a = q_dK_a-2c\sqrt{K_a}   ———- (1)

P_p = q_0K_p + 2c\sqrt{K_p}   ———-(2)

For equilibrium,

Pa = Pp

Or,   q_dK_a-2c\sqrt{K_a} = q_0K_p + 2c\sqrt{K_p}

Or, \dfrac{q_d}{K_p}-2c\sqrt{\dfrac{1}{K_p}} = q_0K_p + 2c\sqrt{K_P}

Or, q_d = q_0K_p^2 + 2c\sqrt{K_p} + 2cK_p\sqrt{K_p}

Therefore, q_d = q_0K_p^2 + 2c\sqrt{K_p}(1+K_p)

q_d = q_0\times N_q+cN_c   ———- (3)

Where,

N_q = K_p^2 and N_c = 2\sqrt{K_p}(1+K_p)

One Thought to “Prandtl and Bell Theory on Bearing Capacity Analysis”

  1. Red Bro
    February 5, 2017 at 12:28 pm

    hello sir can you add the detail regarding the derivation of equation according to prandtl for utimate bearing capacity as well

    Reply

Leave a Comment