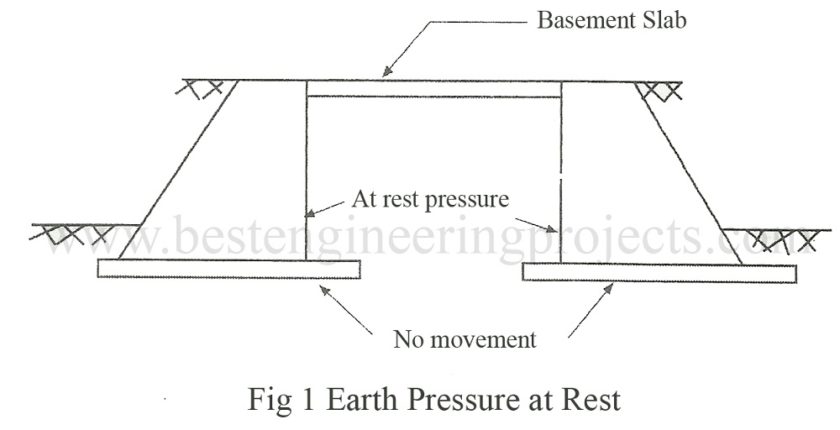
Earth Pressure at Rest | Coefficient Earth Pressure at Rest

When a soil mass gets deposited naturally or artificially, the coefficient of earth pressure attains a value known as coefficient of earth pressure at rest (K₀) which is intermediate between coefficient of active earth pressure (K_a) and coefficient of passive earth pressure (K_p) values. In this case the horizontal stress is given by,

$P_h=f(\sigma_v)=K_0\gamma z$ —————————–(1)

Where, $\sigma_v$ = Vertical Stress at depth ‘z’

$\gamma$ = Unit weight of soil

z = Depth below ground surface

The value of K₀ depends upon the relative density of sand, and the process by which the process of deposition has taken place. If the process does not involve artificial tamping, the value of K₀ ranges from about 0.4 for loose sand to 0.6 for dense sand. Artificial tamping increases the value of K₀ which is about 0.8.

Derivation of earth pressure at rest (K₀)

The value of K₀ can be obtained by the theory of elasticity. Consider a cylindrical sample of soil which is acted upon by stresses $\sigma_x, \sigma_y, and \sigma_z$ , in three principal directions. Let $\epsilon_x$ be the strain induced in the direction of $\sigma_x$ . Then we can write,

$\epsilon_x=\dfrac{\sigma_x-\mu(\sigma_y+\sigma_z)}{E}$ ———————(2)

But in this case, $\sigma_x = \sigma_y\ and epsilon_x =0$

Hence,

$\sigma_x-\mu(\sigma_x+\sigma_z) = 0$

Or, $\sigma_x(1-\mu)-\mu\sigma_z = 0$

Or, $\sigma_x=\left[\dfrac{\mu}{(1-\mu)}\right]\sigma_z$

Or, $\sigma_x=\left[\dfrac{\mu}{(1-\mu)}\right]\gamma z$ ——————————(2.a)

Therefore, $\sigma_x = K_0\gamma\ z$

where, $K_0=\left[\dfrac{\mu}{(1-\mu)}\right]$ —————————-(2-b)

Jacky in 1948 has suggested an approximate relationship for K₀. The relation is:

$K_0 = 1-sin\phi$ ——————(3)

For normally consolidated clay, the value of K₀ is less than 1. For pre-consolidated soils with over-consolidation ratio more than 4.5, K₀ could be 1 or even more. For normally consolidated clays,

Brooke and Ireland (1965) suggested relation for K₀ as:

$K_0 = 0.95-sin\phi$ ——————(4)