Immediate settlement takes place in a short time (about a week) after the application of load and is due to elastic distortion of the soil. As the settlement is experienced in a short time, there will not be enough time for soil mass to change in its water content. Hence, Settlement takes place under constant Volume or under un-drained conditions. Immediate settlement is computed by elastic theory. Compared to consolidation settlement, particularly in saturated clay, immediate settlement is very small and often neglected unless the structure is very important.
Settlement of Footing in Clay
The total settlement is given by:
Where s is the total settlement, si is the immediate settlement, sc is the consolidation settlement and ss is the secondary settlement,
Computation of Immediate settlement
There are various methods of computing the immediate settlement. The most widely used are Schleicher’s method and Jambu, Bjerrum, and Kjaernsli’s method.
Schleicher’s Method – In this method the settlement at the corner of the rectangularly loaded area at the surface of a semi-infinite medium of a homogeneous and isotropic soil mass is given by:
———- (1)
Where, si = Immediate settlement
q = Load on foundation
B = Smaller dimension of the loaded area
= Poisson’s Ratio
Is = Influence coefficient
E = Modulus of elasticity
To the settlement at the center, the principle of superposition is used. For a rectangular area, the settlement at the center is equal to four times the settlement at the corner.
The Value of the influence factor Is for a saturated clay layer of semi-infinite extent can be obtained from Table 1.
Table 1 Values of Influence Factors (Is)
|
The shape of Loaded Area |
Flexible |
Rigid |
||
|
Centre |
Corner |
Average |
||
|
Circular |
1.00 |
0.64 |
0.85 |
0.80 |
|
Rectangular (L/B) |
||||
|
1.0 |
1.12 |
0.56 |
0.95 |
0.90 |
|
1.5 |
1.36 |
0.68 |
1.20 |
1.09 |
|
2.0 |
1.53 |
0.77 |
1.31 |
1.22 |
|
5.0 |
2.10 |
1.05 |
1.83 |
1.68 |
|
10.0 |
2.52 |
1.26 |
2.25 |
2.02 |
|
100.0 |
3.38 |
1.69 |
2.96 |
2.70 |
Rigidity Correction – The rigidity correction is done by multiplying the flexible settlement obtained from the equation above by a factor, 0.8 called the Rigidity Factor.
Alternatively we can write:
———- (2)
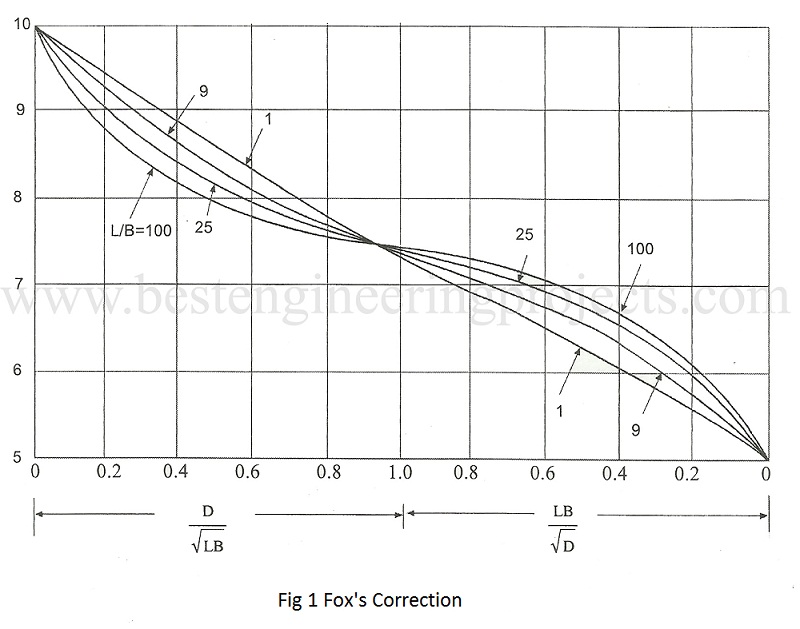
Depth Correction – A foundation placed at the surface will settle more than a foundation placed at a certain depth. To account for this, Fox 1948 has given a chart for depth factor, which is shown in Fig.1.
Significant Depth – The depth at which the additional stress imposed by the foundation to the sub-soil becomes negligible is called the significant depth. This is generally taken as the zone bounded by a 20 % pressure bulb corresponding to which the depth is 1.5 times the width for a square foundation and 3 times the width for a strip foundation. For important structures, the zone bounded by 10 % contours is taken as the significant depth. Corresponding to this decrease in vertical stress, the significant depth for the square foundation is taken as 2 times the width and for the strip foundation 6 times the width. For rectangular foundations, the shorter dimension should be used to decide upon the significant depth.
Janbu, Bjerrum, and Kjaernsli’s Method Janbu, Bjerrum, and Kjaernsli (1956) have suggested the equation to compute average immediate settlement under a flexible compressible foundation in saturated undrained clays. The equation is given below:
———- (3)
Where, si = Average immediate settlement for flexible footing
µ0 µ1 = Shape factors obtained from the charts shown in Fig.2
Eu = Undrained modulus of elasticity of the soil
q = Load on foundation
B = Smaller dimension of the loaded area
How can we findout magnitude of immediate settlement and on what factors it depends ?
What is the thumb rule for settment of earth in an uncompacted embankment