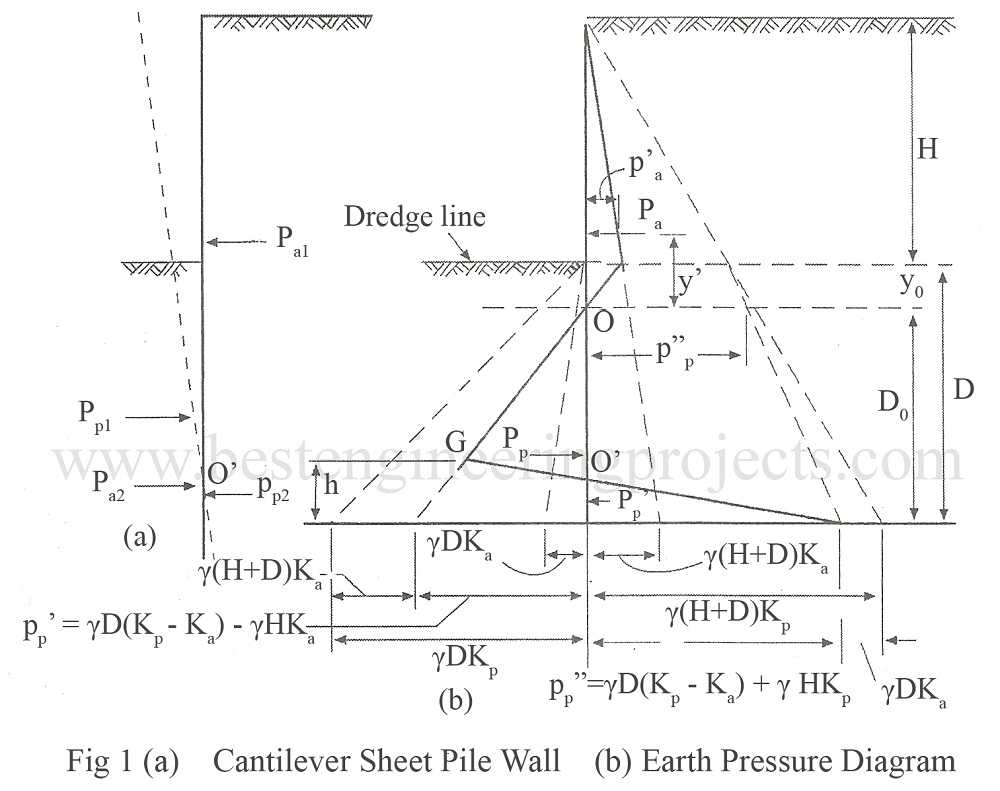
A cantilever sheet pile is shown in Fig.1 below. The Fig,1 also shows the pressure distribution.
When the wall rotates about the point O’, the various pressures acting on the wall are:
Pa1 = Active earth pressure on the back of the wall above the point O’
Pa2 = Active earth pressure in front of the wall below the point O’
Pp1 = Passive earth pressure in front of the wall above the point O’ up to dredge line
Pp2 = Active earth pressure on the back of the wall below the point O’ In the derivation the various notations used are:
D = Minimum depth of embedment with a factor of safety equal to l
Ka = Rankine active earth pressure coefficient
Kp = Rankine passive earth pressure coefficient
K = Kp – Ka ———- (1)
pa‘ = Effective active earth pressure acting against the sheet pile at the dredge line level
pp‘ = Effective passive earth pressure at the base of the sheet pile and acting towards the backfill
Pp” = Effective passive earth pressure at the level of O’
= Effective unit weight of the soil assumed same below and above dredge level
y0 = Depth of point O below the dredge line where the active and passive pressure are equal
y’ = Height of point of application of the total active earth pressure Pa above point O
h = Height of point G above the base of the wall
D0 = Height of point O above the base of the wall
Expression for y0
At the point O, the passive pressure acting towards the right should be equal to the active pressure acting towards the left, ie.,
———- (2)
———- (3)
Or,
From Eqs.(1) and (3)
———- (4)
Expression for h
For equilibrium, the algebraic sum of all the forces in the horizontal direction is zero. Thus,
———- (5)
Or, ———- (6)
Taking moments of all the forces about the bottom of the pile, and equating to zero we have,
———- (7)
Substituting the value of h from Eq.(6) into Eq.(7), get the value of D0. The method of trial and error is generally adopted to solve the equation. The minimum depth of embedment D with a factor of safety equal to 1 is therefore,
D = D0 + y0
To obtain a minimum factor of safety, the depth D shall be increased by 20 to 40 %.