Cantilever Walls in Cohesive Soils - Engineering Projects

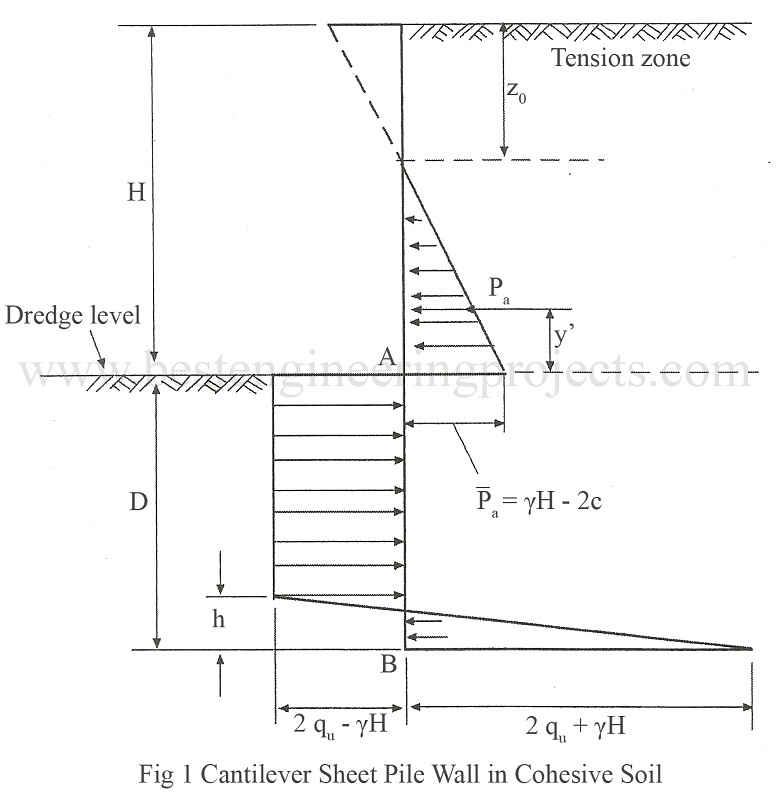
The pressure distribution on a cantilever wall constructed on cohesive soil is shown in Fig.1 below.

The active pressure at any depth z can be expressed as:

$p_a = \sigma_v \times K_a-2c\sqrt{K_a}$

Where,

$\sigma _v$ = Vertical pressure, $\gamma Z$

Z = Depth from surface to backfill

The passive pressure at any depth y below the dredge line may be expressed as:

$p_p = \sigma_v \times K_p + 2c\sqrt{K_p}$

The active earth pressure on the wall from the backfill surface to the dredge level is shown in the Fig.1. The soil is in tension up to a depth of z₀ and pressure in the wall is zero in this zone. The net pressure distribution on the wall is shown by the shaded triangle.

The active pressure acting towards the left is given by:

$p_a' = \gamma H\times K_a-2c \times \sqrt{K_a}$

When, $\phi = 0, P_a' = \gamma H-2c = \gamma H-q_u$ ———- (1)

Where, q_u = Unconfined compressive strength of clay soil = 2c

The passive resistance acting towards the right at the dredge level can be expresses as:

$p_p' = 2c = q_u, When, \phi = 0$ ———- (2)

The resultant of active and passive pressures at the dredge line is given by,

$p_p'-p_a' = 4c-\gamma H = 2q_u-\gamma H$ ———- (3)

The resultant of active and passive pressure at any depth y is given by,

$p_p = \gamma y + q_u$

$p_a = \gamma (H+y)-q_u$

The resultant of active and passive pressures at any depth is now can be expressed as:

$p_p-p_a = \gamma y+q_u-\gamma(H+y)-q_u$ ———- (4)

$p_p-p_a = 2q_u-\gamma H$ ———- (5)

The above Eqs.(4) and (5) indicate that the resultant pressure remains constant at all depths. The passive earth pressure developed on the backside of the wall at the bottom of the pile can be expressed as:

$p_p = \gamma (H+D) + q_u$ acting toward left ———- (6)

$p_a = \gamma D-q_u$ ———- (7)

The resultant of active and passive pressures at the bottom of the Wall can be expressed as:

$p_p-p_a = \gamma (H+D) + q_u-\gamma D + q_u$

$p_p-p_a = 2q_u + \gamma H$ ———- (8)

For equilibrium, the sum of all horizontal forces should be equal to zero. Then,

$p_a-(2q_u-\gamma H)D + \dfrac{1}{2} \times 4 \times q_u \times h = 0$ ———- (9)

The Value of h can be calculated from the Eq.(9). For equilibrium, the sum of moments of all forces at base also should be equal to zero. Then,

$p_a(y'+D) + \dfrac{h^2}{3}\times 2q_u-(2q_u-\gamma H)\dfrac{D^2}{2} = 0$ ———- (10)

Substituting the value of h obtained from Eq.(9) into Eq.(10), we can get the depth of penetration D. The depth so computed should be increased by 20% to 40% so as to have a factor of safety of 1.5 to 2. Alternatively, the unconfined compressive strength can be divided by a suitable factor safety.

Share this:

Leave a Comment Cancel reply