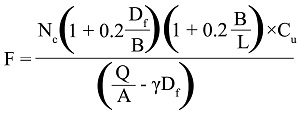Bearing Capacity of Mat Foundation

Engineeering Projects

What is Bearing Capacity of Mat Foundation?

Cohesionless Soil – In cohesionless soil a mat foundation does not fail in shear. For example, the bearing capacity of a raft foundation having a width of 6 m, and founded to a depth of 3 m in a sand deposit having relative density corresponding to SPT N-value of 10 is about 700 kN/m2 which is extremely high. In such case, the foundation may experience excessive settlement or even fail before attaining a bearing pressure of the order cited above. This means that the bearing capacity of raft foundation in sands is governed by settlement except for the case where the sand deposit is very loose i.e. the corrected SPT value is less than 5.

Most of the structures in sands can tolerate a differential settlement of about 3/4 of the maximum permissible settlement. This fact has been the basis for the design of shallow footings in sands. Compared to spread footings the differential settlement in a mat foundation is much more reduced as the mat bridges over the loose pockets and lenses of a soil deposit. It has been found that, for the same loading intensity and soil condition, the differential settlement in a mat foundation is about 1/2 than that of a spread foundation. This fact suggests that the maximum settlement or healing pressure in the case of the mat foundation can be taken as the double of the value assigned to the spread foundation.

As per Teng (1962) the safe bearing capacity can be obtained from the results of SPT test.

From shear failure criteria, the net safe bearing capacity is given by:

qns = 0.02N2BRW1 + 0.06(100 + B2)DfRW2   ———-(1)

From settlement criteria of 25 mm the safe bearing pressure is,

qna = 1.75(N-3)RW1   ———-(2)

Where, B = smaller dimension of the footing

Df = Depth of foundation

N = corrected SPT Value

RW1, RW2 = water table correction factors

Where, qns and qna are in ton/m2

It has been found that the Teng’s equation for bearing capacity based on allowable settlement is conservative and hence it is suggested to use the relation suggested by Peck et al (1974). The relation is:

qna = 22RWN   ———-(3)

The above equation is applicable for 5 \leq N \leq 50. If the value of N after proper correction is less than 5 the raft would not be feasible. The sand is either compacted or a deep foundation is provided. For N values greater than 50, the above equation gives conservative results. The value of qna is for 50 mm allowable settlement, for other values of allowable settlement a linear interpolation is to be done.

Cohesive Soil – In cohesive soil the net ultimate beating capacity on the basis of shear failure criteria is determined by Skempton’s equation, which is:

 cohesive soil  ———- (4)

Where, Nc is the bearing capacity factor for a strip footing at surface and is taken as 5. Cu is the undrained shear strength, Df, B and L are depth, width and length of footing respectively. The safe bearing capacity is given by:

q_{ns} = \dfrac{q_{nu}}{F}   ———-(5)

Where, F is the factor of safety. The factor of safety should neither be less than 3 for normal loading condition and 2 for extreme loading condition.

The quantity in the above equation is the pressure at the elevation of the base of the foundation in excess of that exerted by surrounding surcharge. If Q be the total load imposed at the base of the raft due to dead load and live load, the gross pressure at the base is Q/A , Where A is the area of the raft.

In terms of net bearing capacity the gross pressure is,

\dfrac{Q}{A} = q_{ns} + \gamma \times D_f   ———-(6)

Where \gamma is the unit weight of the soil and Df is the depth of foundation. Thus we can write the net allowable bearing pressure as:

q_{ns} = \dfrac{Q}{A}-\gamma \times D_f   ———- (7)

Hence the factor of safety can be written as:

 conventional method 3  ———- (8)

 

If a raft is placed at depth Df such that the weight of excavated soil becomes equal to the gross applied load, the factor of safety becomes infinite i.e. \dfrac{Q}{A}-\gamma D_f=0. In such a case the raft foundation is called a “Floating Foundation”, where the total load at the base of the raft is fully compensated by the weight of the soil excavated. However, in practice it is not possible to obtain a fully compensated raft possibly due to the uncertainties involved in the estimation of the loads or in the action of surcharge.

The factor of safety does not decrease proportionately with the increase in load. Compared to rate of increase in load, the decrease in factor of safety is very rapid. Furthermore, an error in estimating the loads (LL + DL) has a greater influence in the factor of safety for a weak soil than for a strong one.

The depth of foundation for a raft to obtain a desired factor of safety is a trial and error procedure. However, the convergence is very rapid if a reasonable value of D and B is assumed.

Leave a Comment